pH in Rain An environmentalist wanted to determine if the mean acidity of rain differed among Alaska, Florida, and Texas. He randomly selected six rain dates at each of the three locations and obtained the following data:

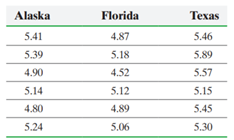
(a) State the null and alternative hypotheses.

(b) Verify that the requirements to use the one-way ANOVA procedure are satisfied. Normal probability plots indicate that the sample data come from a normal population.

(c) Test the hypothesis that the mean pHs in the rainwater are the same at the  level of significance.

(d) Draw boxplots of the pH in rain for the three states to support the results obtained in part (c).

A large manufacturing company produces computer printers that are distributed and sold all over Europe. Due to lack of industry information, the company has a difficult time ascertaining its market share in different parts of the continent. They hire a market research firm to estimate their market share in a northern country and a southern country. They would also like to know whether there is a difference in their market shares in these two countries; if so, they want to estimate how much. The market research firm randomly selects printer customers from different locales across both countries and determines what brand of computer printer they purchased. The following Minitab output shows the results from this study. Discuss the results including sample sizes, estimation of the difference in proportions, and any significant differences determined. What were the hypotheses tested?